Simulating Simple World-Systems:
Power and Hierarchy in Intersocietal Networks*
Jesse Fletcher, Jake Apkarian, Kirk Lawrence, Hiroko Inoue,
Christopher Chase-Dunn, and
Robert Hanneman
August 13, 2010
Words: 8,229
Institute for
Research on World Systems (IROWS)
University of California, Riverside
*This research has been supported by National Science Foundation grant NSF-HSD SES-0527720. The communicating author is Robert Hanneman (robert.hanneman@ucr.edu). It is IROWS Working Paper # 63 available at https://irows.ucr.edu/papers/irows63/irows63.htm
Abstract: World-Systems theory argues that societies need to be studied at the system level of analysis. The complexity and scale of human societies is related to their positions within large, sometimes global, intersocietal networks. By building on ecological predator-prey models, we have generated simulations of an evolving system of societies linked by migration, warfare, and economic trade. A one-dimensional system of societies in which the technology of each society evolves in response to selection pressures arising from conflict with its neighbors, starvation from lack of resources, and technological diffusion is used to demonstrate the applicability of network analysis to intersocietal phenomena. The models suggest that the interactions among multiple societies in a simple topology can produce complex emergent outcomes. They demonstrate how the regular and structural positions of a society in an intersocietal network are influential in determining the relative success and power of the society in the larger system of societies.
Keywords: world systems, networks, demographics, warfare, migration, trade, technology, simulation
The Dynamics of World-Systems
Theories of historical change identify processes at the level of single systems in relative isolation (e.g. Parsons 1982), as well as processes of interaction between social systems that may give rise to emergent patterns of organization at the global scale (Chase-Dunn and Grimes 1995). World-systems theory (WST) claims that the interactions occurring within “intersocietal networks” are important determinants in the distribution of resources and ultimately power in said networks (Chase-Dunn and Hall 1997: 28). The movement of people, resources, and conflict can influence the scale and complexity of human settlement systems. Historical and archaeological evidence has shown that the behavior of these systems can be exceedingly complex; at times they may appear highly chaotic, and at other times they may display great stability and order. Some systems contain a single hegemony, others a bi-polarity, and still others a core/semi-periphery/periphery hierarchy.
Researchers studying the complex dynamics of coupled natural and mechanical systems (e.g. Strogatz and Stewart 1993; Watts 1999a; Watts 1999b) have been fascinated by the emergence and dissolution of synchronized change. Complexity theorists have produced formal models of how local and closely-coupled interactions in large populations of actors sometimes give rise to varying forms of synchronic movement at the level of the entire population or macro-regions of it. Perhaps particularly notable in the complex systems research tradition is work that explores the emergent dynamics of coupling together local systems that, themselves, have internal dynamic processes.
Network exchange theories along with Elementary Theory (ET) in social psychology (Cook, Cheshire, and Gerbasi 2006; Willer and Emanuelson 2006) study the emergent properties of network structures and how structural topology is a determinant of power distribution within a network. Insofar as societies studied at the system level are said to exist within an “intersocietal network, it is conceivable that many of the laws governing micro-level network dynamics of power translate to the macro-level world-systems analysis of intersocietal networks.
In this paper we explore how research in macro-historical sociology might be informed by adapting the formal approach of conceptualizing societies ascomplex systems. Furthermore, we introduce the elements of network analysis by coupling these complex systems together across the uni-dimensional array of a 5-line network. To accomplish this, we first develop a single-society system. This initial starting point is based on well-established models of ecological predator-prey interaction that have been further modified to better characterize the special features of human societies at very small scale and low technology.
We then couple two such single-society systems together by the movement of people (migration), resources (trade), and conflict (warfare) and explore the resultant emergent behavior. Next, we create the simple complex system composed of five societies (the 5-line network of network exchange theories) also interacting through migration, warfare, and trade. Finally, in an effort to emphasize and understand the importance of resource ecology on these emergent dynamics, we explore how different resource distributions across the line topology affect the behavioral dynamics of these simple societies. The parallels between our models of intersocietal networks and networks studied by network exchange theories and ET are explored.
World-Systems and Evolutionary Theory
Theories of the long-term evolution of societies identify competition and conflict within and between societies as major selection forces. Variations in form (which may be retained or not, based on selection) arise from endogenous invention, but also from the diffusion of culture that follows from migration and prolonged interaction among populations. Both of these core ideas implicate interactions among human populations as the driving force behind macro level change.
Oddly, most systematic sociological theories of long-term social change do not explore the effects of the interactions between macro-actors with the same rigor that they apply to endogenous dynamic processes. Parsons (1982), for example, argues strongly for the role of selection pressures driving change in productive, military, and organizational technology. His vision of these dynamics, however, is largely limited to the needs of a single social system (i.e. society) viewed in isolation. Spencer (Spencer and Carneiro 1967) is more explicit in arguing that warfare between populations acts as a selection pressure, but goes no further in exploring the dynamics of this process; the view adopted is that of a society nested within a generalized (often conflictual) environment.
Ecological theory (primarily from anthropology) has been more explicit about the dynamics of populations of human societies. Tainter (1988) and Carneiro (1970) particularly suggest that population pressures in single societies may lead to territorial expansion, reducing internal selection pressures. Both note, however, that systems of societies tend to ultimately exceed the carrying capacity of their environments, leading to warfare among the settlements and/or the emergence of hierarchy and/or collapse. These theories, however, are not formal. At best they suggest a range of possible outcomes in the dynamics of multi-population systems.
The theory that guides the development of our formal model, Hall and Chase-Dunn’s iteration theory of world systems evolution (Chase-Dunn 2001; Chase-Dunn and Hall 1997) borrows heavily from the ecological theories. The iteration theory is more explicit about how the growth of human populations in a circumscribed space may generate selection pressures for the emergence of greater complexity within societies, as well as the emergence of various forms of order among multiple societies. The iteration theory identifies key dynamic processes at an abstract level, but does not deal explicitly with complex dynamics.
Historical research on the evolution of systems of human societies, in contrast to sociological theory, often identifies complexity (though not by that name) as critical in macro-dynamics. Historical explanations give great weight to initial conditions, and emphasize how the overall evolution of systems may display path dependent development (e.g. Kottak 1972; Maloney 2008; Tagliacozzo 2007). Importantly, the interacting human populations in most historical explanations are usually quite heterogeneous – varying in culture, technology, and geography.
We will instantiate the iteration theory of evolution of world-systems of societies in a simple complex system model. This approach applies the evolutionary and ecological rules from received theory, but applies them in a (minimally) complex spatial topology of five societies (a simple “line” network).
Power Distribution, Network Topology, and Complexity
Work on power and exchange in social networks (Willer 1999) strongly suggests that the topology of the connections among social actors can exert major influence on the distribution of power and resources in the network. For example, in exclusively connected 5-line exchange networks, the most central actor and most peripheral actors (actors on the edges of the network) may be exploited by the interstitial actors who have the advantage of possessing exchange partners that are solely dependent on them (whereas the others do not have access to exchange partners with no alternatives). This type of network is known as a strong power network where the power of the peripheral actors is much less than the power of the central actor who is less powerful than the interstitial actors. Exclusion from exchange is the key determinant of power distribution in these types of networks.
Research in complex systems (coupled oscillators, network embedded game theory, etc.) explores interactions among large numbers of agents, in varying (and sometimes evolving) topologies. In complex systems, as the agents become more adaptive and their behavioral rules more complex, the range of emergent system states that may exist, their stability, and dependence on chance and initial conditions increase dramatically. Our model attempts to incorporate some of the complexities that network exchange theorists are only beginning to explore including adaption and feedback.(Walker, et al. 2000).
Complex systems and network exchange research, however, usually pay little attention to agent heterogeneity that is a fundamental characteristic of the interactions among multiple human populations existing in geographical space. One of the primary ways that actors can be (and often are) differentiated in exchange networks are through their relative levels of the resources they possess. Some actors in a network may be resource rich, while others may be relatively resource poor. In most network exchange models, however, resource production and/or distribution is either exogenous or forced to be homogeneous across actors. We briefly explore the interplay between network topology and the effects of differentiating enrichment levels of societal nodes in the network.
The Model
Each simulation model describes the interplay of population size and natural resources in simple human settlements that evolve greater organizational and production technology over time. Initially the population occupies a local catchment area, but as that area fills up pressure will begin to build for the society’s population. The population-resource dynamics of the local society eventually generate population increases that strain the local resource environment. This leads to waves of migration from the local society to the surrounding region. As both the local area and the surrounding region approach carrying capacity (circumscription), it becomes more difficult to migrate outward. Eventually, migration will cease to be a viable response to pressures from resource shortage and over-crowding. When this occurs, warfare emerges between the local society and the recently emergent societies in the larger surrounding area.
In the simplest of the models presented here, warfare and trade do not occur, because the simulation is of a single society living in isolation. In the largest models presented here, five local societies are linked through warfare, trade, and migration in a line topology. In these models, a small population begins in the center of the line with two unpopulated regions spread out on either side. As the center society fills up, it can migrate out into the surrounding regions, which will eventually fill up and begin to react back on the original society through the additional flows of warfare and trade.
The Individual Society
Each society consists of a human population living and exploiting the resources of a catchment area of fixed size and potential productivity. An individual society is essentially a consumer-resource model built with coupled ordinary differential equations that regulate human and resource population flows. We’ve based our model on current ecological predator-prey models that have evolved from the seminal Lotka-Volterra equations. The general form of the equations governing the human and resource population dynamics in a single society are:
![]() (1)
(1)
![]() (2)
(2)
In these equations, R is the resource population and P is the human population.
In the resource growth rate equation (1), environmental degradation (soil erosion, pollution, etc.) reduces the rate at which natural resources recover. This is assumed to be a function of population size and is captured in the resource growth rate equation by e(P). All else being equal, resource populations (as well as human populations) are assumed to reproduce at a normal birth rate, which is a constant represented as nRb (or nPb for humans). R0 is the initial resource population level. K is the carrying capacity of the resource population which cannot grow unbounded and is assumed to max out due to land constraints. The MIN function is the rate at which resources are harvested for consumption which is dependent on both human and resource populations. The harvesting rate is influenced by a process known as intensification. Intensification is defined as “the investment of more soil, water, minerals, or energy per unit time or area” (Harris 1977: p. 5). As the population of a society increases, and resources become more and more scarce, individuals must increase their resource gathering efforts. Intensification is captured in the harvesting multiplier h(P) and is a logistic function of human population density (which is directly proportional to P). The model assumes that increasing population densities make for less efficient resource extraction due to decreasing marginal returns to increased labor effort. The amount of resources harvested is the smaller of h(P)∙P and R. Certainly, if R < h(P)∙P only R number of resources are available to be harvested.
In the population growth rate equation (2), nPd is the normal human death rate constant. When all else is equal, this model makes the Malthusian assumption that births will slightly exceed deaths, but that the realization of these processes is stochastic. The “predator functional responses” (Berryman 1992) that govern human births and deaths based on human-resource interaction, b(R/P) and c(R/P), are ratio dependent and a function of consumption levels within the society. Migration from the local society to the surrounding region is driven by high levels of internal conflict, and by poor material conditions (both of which are results of low per capita consumption due to high population density and population pressure in the local society). Migration out of the society is a complex function of consumption levels and captured by the term m(R/P).
The resource reproduction rate governs how many new resources are added to the environment in each yearly cycle. This corresponds with the environment’s ability to “re-grow” or recover from the prior cycles of resource harvesting. We suppose that the rate at which resources are renewed is constant and asymptotically approaches the carrying capacity. This is based on Turchin’s (2003) “regrowth” function for vegetation and small game resources. This marks one major deviation from most current ecological predator-prey models that assume logistic prey growth.
The local population extracts resources from the environment at a rate that assumes, under normal conditions, that every individual will harvest slightly more resources than those necessary to sustain themselves (if they are available). When the simulation begins, the model assumes that all resources which are extracted are consumed. However, the ability to store resources can be “learned” by each society as their technology increases and surpasses a certain threshold. However, in the early stages of the model there is an inherent tendency for per capita consumption to rise as people consume the extra resources they have gathered. This, in turn, leads to increasing rates of population growth and higher population density. Increases in population density, in turn, modify the population’s effects on the resource environment by intensification and degradation.
Technology in the single society grows over time as a function of starvation deaths. As more societies are added to the model, technology will also increase as a function of deaths accrued from warfare. Evolutionary theories suggest that societies may adapt to selection pressures exerted either by the consequences of reaching carrying capacity in their environment, or by conflict with other societies (Spencer and Carneiro 1967). Adaptive responses may take the form of more complex and productive technologies of various sorts: human capital, material technologies, and social technologies (esp. hierarchy and solidarity that contribute to greater coordination of social agents) (Chase-Dunn and Hall 1997). Additionally, technology can spread between societies that have frequent and systemic contact with one another, a process known as technological diffusion (Barro and Sala-i-Martin 1997).
Technology (T) affects the relationship between a society and its resource base by increasing the productivity of resource extraction (h(P)). It also affects the relationship between each society and its neighbors by increasing the military force it can project (force is a function of the product of P and T). Societies which are able to project greater force are also more able to exploit their neighbors by threat of violence, and in extreme cases establish a “tributary empire” whereby all warfare between the stronger and weaker society ceases and the weaker society pays tribute in the form of all excess resources not needed for survival. In short, direct political control is obviated in favor of simple economic extortion, an event common in the historical and archaeological history of human societal evolution (Bang 2003). Last, technology also allows society to “invent” resource storage. Once technology increases beyond a uniform (but arbitrary) threshold, the local society is able to move any resources not necessary for subsistence into storage. Stored resources can be consumed if harvesting rates fall below subsistence levels, or can be “traded” with other societies.
Solomon and Holling argue that successful logistic predator-prey (or consumer-resource) models must assume that prey death rates are a nonlinear function of prey density due to effects like saturation (predators do not always consume prey every time they interact) (Berryman 1992). They introduce nonlinear response functions into their models that modify prey death rates and predator growth rates as predator and prey interact. Arditi and Ginzburg claim that these nonlinear response functions act on faster time scales than the rest of the model, and that if interaction is modeled as the ratio of predator and prey density rather than the product, this issue can be fixed (ibid). Predator-prey models that employ the use of ratio response functions generate exclusively stable steady state solutions. In our SSDR model, the predator (human) growth rate equation uses nonlinear response functions that are ratio dependent and are therefore consistent with current ecological models. Though our models use a different functional form for prey growth, we would expect stable steady state solutions similar to the logistic models (Berryman 1992).
Intersocietal Dynamics
A system of local societies is composed of discrete populations linked by three processes: migration, trade, and warfare. The first two of these processes affect the movement of individuals and resources across space, while the third exerts pressure on societies to increase their level of technology. Furthermore, migration and warfare work to reduce population pressures in the each society by reducing the number of individuals in that society. Trade benefits societies by either a) facilitating the acquisition of additional resources from weaker societies (thereby increasing consumption per capita), or b) avoiding warfare deaths incurred by stronger societies.
In our 2-line and 5-line models, migration is inhibited by circumscription in the adjacent societies; as they attain high population densities, the pressures to migrate from the local society must become greater and greater for a migration to occur. At high enough levels of circumscription, migration from the local society to the adjacent societies is halted.
Circumscription results when natural increases in the size of a local society and migration from a neighboring society combine to create high population densities and restricted life chances for all individuals in the local society. As population densities in two adjacent societies increase beyond carrying-capacity levels, both societies will experience extreme pressure to migrate, but neither society will have an appropriate outlet with which to do so. When this occurs, circumscription increases at an exponential rate. To the degree that consumption per capita is below subsistence levels, the rate of increase is even more rapid. High levels of circumscription increase the likelihood of warfare between societies.
Warfare between the societies of the regional world-system is initiated at rates proportional to rates of contact between the populations of the local society and the populations of the other societies in the regional world-system, and increases as circumscription increases. Contact among the two populations is directly proportional to the product of the population sizes. Below a threshold point of circumscription, contact between the two populations does not result in conflict. However, as the average circumscription evidenced in the two societies grows, populations are increasingly unable to move to alternative locations when confronted with outsiders. The rate of conflict initiation (warfare), then, is proportional to the product of the two population sizes and the level of circumscription between the two societies. Once initiated, conflict decays exponentially as combatants are exhausted and/or grievances negotiated.
The number of people that die from warfare is modified by the population and technology ratios of a society versus its war partner. A society can be especially effective on the battlefield if they can field an especially large army compared to their opponent. Also, a society can excel in warfare insofar as their technology (e.g. organizational, material, logistical, etc.) is superior to their opponent’s. Warfare advantage is determined by the product of these ratios. When warfare advantage crosses a critical threshold, the society with the higher advantage establishes the aforementioned “tributary empire” with the other society, suspending war in exchange for all of the weaker society’s excess resources.
Trade occurs when societies have stored resources that they are able to exchange with one another. Once storage technologies have been invented between two neighboring societies, they will under normal conditions attempt to trade their stored resources. The terms of trade are determined by the relative warfare advantage between the two societies.
The more coercive force a society can apply to its neighbor, the more beneficial the trade relations with its neighbor will be. Recall that warfare advantage is a function of population size and technological advancement. Similarly, trade advantage is a function of the product of the population and technology ratios between the two societies. If the product of these ratios equals one (implying that the two societies have equal military force), the trade relations between the two societies are equal (i.e. one resource is traded for one resource). When warfare advantage is not equal, the society with more advantage nets a larger amount of the combined resources each iteration.
In our model, with homogenous, indistinguishable resources, trade does not resemble exchange processes. If Society A has a warfare advantage equal to 1.25, Society B’s advantage is 1/1.25 = 0.8. The amount of resources each society gets from the other is a function of their warfare advantage. So the net effect is a transfer of resources from B to A. The threat of force by A is what drives this net transfer. This is very similar to the coercive relations described by Elementary Theory. The threat of a negative sanction from A causes the flow of a positive sanction from B. As Willer states, “in coercion, typically only one sanction flows” (1999: 27) and that is why we see a unidirectional transfer of resources. The exploitation of one society by another due to unequal terms of trade enforced by threats of violence is commonplace in human history (Chase-Dunn and Hall 1997).
Eventually, the warfare advantage threshold discussed earlier is crossed whereby the society with greater advantage becomes the extorter. When this occurs, it is assumed that the subjugated society has greatly increased contact with their stronger neighbors, as the neighbors must continually return to the local society to extort their resources. This constant exposure leads to technological diffusion. Along with starvation and warfare deaths, technological diffusion is the final means through which technology can grow. During this process, the weaker society will be exposed to the technology of the larger society, and will slowly adopt this technology. This is roughly equivalent to the semi-peripheral advantage discussed in world systems theory, whereby weaker societies have access to the technological innovations of the stronger societies, especially as contact between the two societies increases (Chase-Dunn 2001).
Results
Single Society Dynamics
To better appreciate the dynamics that arise as a result of the coupling of societies, it is useful to first examine a society in isolation. Our model adds some minor complexities to ecological predator-prey models by supposing that humans are able to extract resources at a higher rate from the environment by intensified effort when over-population threatens consumption. As it develops more technology, eventually learning to store surplus production (and developing the social technologies of hierarchy or solidarity to enforce saving).
Most available anthropological evidence shows that most early sedentary human populations are primarily exploiting vegetable and small game resources, which follow a “regrowth” response when consumed (Turchin 2003). This type of resource regrowth tends to avoid the oscillations normally found amongst traditional ecological models of predator-prey dynamics, and instead generates a characteristic smooth movement toward equilibrium in the population. This fact along with the use of ratio dependent response functions to model interaction leads us to expect stable equilibrium solutions.
Figure 1 shows a typical solution of our single-population model. The short-term variation (i.e. noise) is due to the stochastic assumptions about variation in environmental recovery rates (e.g., rainfall fluctuations), and random variation in the human population growth rate due to the normal birth and death constants (which are normally distributed), and migratory responses which are modeled stochastically as well.
Figure 1: Single Society Population and Resource Dynamics
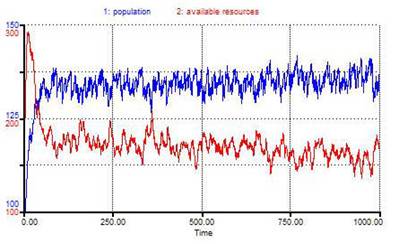
As expected, a single society in isolation tends toward population equilibrium under all circumstances. Over time, this equilibrium level rises as technology increases (as the result of innovation in response to periodic resource shortages). The equilibrium is maintained by a combination of fertility, productivity, and mortality regulation. Importantly, though, stability is also maintained by out-migration. This occurs because of pressure placed on the society due to increasing population density and the resultant internal conflicts over resources. In the single society model, emigrants simply disappear. But in real societies, emigrants move to adjacent niches, eventually filling all available space, and removing migration as a possible regulatory response. Thus, in our single society model, migration is a major determinant of demographic regulation. In intersocietal networks, however, warfare emerges as the prominent demographic regulator.
Intersocietal Dynamics: Two Societies
Once an adjacent society is added to the model, the stable solutions found in the single society model decay into cyclical solutions. The speed at which they decay depends on the ratio of resource carrying capacity (K), or “enrichment level”, to the amount of land in each society. At low values of this ratio (which we will refer to as ρ), the solutions have negligible levels of warfare and the population levels remain at equilibriums of equal value for thousands of iterations. This period is a period of equality where neither of the societies has a warfare advantage and the population levels of both societies are the same. However, at some point, the balance is broken and the population levels begin to oscillate with one society dominating in terms of size and warfare advantage followed by the other and back again. Eventually the system re-stabilizes into a two society hierarchy with one of the societies gaining a warfare advantage that cannot be challenged.
As the level of enrichment in the system grows relative to the amount of land (ρ increases), the point at which the system destabilizes occurs earlier and earlier. Interestingly, a similar phenomenon known as “the paradox of enrichment” was discovered in some ecological predator-prey models (Rosenzweig 1971). When varying the carrying capacity of the prey (resources), as a certain threshold is crossed, the solutions destabilize. Steady state equilibrium solutions transition into oscillating boom and bust cycles. Though the solutions to our model do not involve a phase transition from stable solutions to unstable solutions as the parameter ρ is varied, the point of destabilization is certainly a function of enrichment levels (prey/resource carrying capacity) with the onset of destabilization and intensity of warfare increasing with enrichment. It is possible that in our model, technology drives destabilization. When the environment has very few resources available, the population is regulated by starvation. Overcrowding is not usually an issue in harsh climates (deserts, tundra, etc.). However, as technology allows for higher consumption levels in the societies and the carrying capacity rises, higher rates of contact between societies could lead to the destabilization of the system and to cycles of warfare.
Interestingly, there is a critical value of ρ (call it ρc) at approximately ρ = 1.5 such that whenever ρ > ρc, the solution starts in a destabilized state. When ρ < ρc, and the solutions start at equilibrium (Figure 2), the society that comes out on top is entirely decided by random processes giving either society a 50-50 chance at ultimately becoming the stable hegemon (most powerful node), regardless of the initial population levels of the societies. However, when ρ > ρc (Figure 3), the initial population levels suddenly become important in establishing hegemony. If the initial population levels of the two societies are equal, like in the case where ρ < ρc, the hegemon is determined randomly. However, when one society starts with a significantly greater number of individuals than the other and the smaller society is below its carrying capacity, the society that started with fewer individuals always becomes the more powerful society.
Figure 2. Intersocietal 2-Line Model with ρ = 0.95
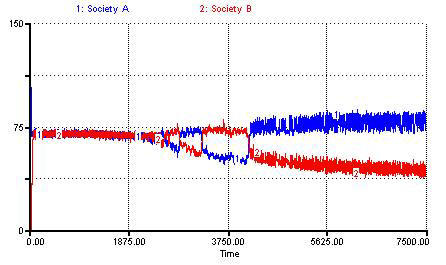
Figure 3. Intersocietal 2-Line Model with ρ = 2
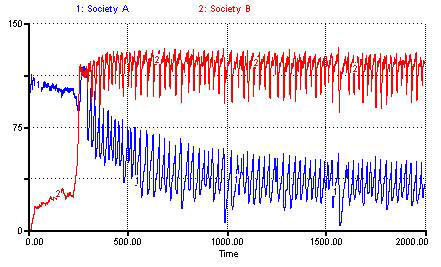
As an example of why this happens, assume Society A starts with a significantly higher population level than Society B (roughly two times or greater) and that they share similar levels of technology. Society A starts out with a warfare advantage and initially dominates Society B exploiting it for excess resources while killing a greater proportion of Society B’s populous in battle. This causes the technology level of Society B to grow at a much faster rate than the technology of Society A. However, because Society B is below carrying capacity, natural growth along with individuals migrating from Society A (due to greater levels of population pressure in A) cause the population of B to grow at a faster rate than A as well. By the time the population of B approaches that of A, it has a significant technological advantage and shortly overtakes A in population size. This massive swing in the momentum of warfare advantage (where for a time B controls both a population and technological advantage) gives B the push it needs to establish itself as the stable hegemon of the system. Though Society A’s level of technology will eventually catch and overtake that of Society B, it can never achieve enough momentum in overall warfare advantage to ever regain hegemony.
Once the stable hierarchical solution is established, the population levels of the two societies do not reach stable equilibrium values. They oscillate in vicious cycles of warfare. As the warfare advantage of Society B (the hegemon) grows higher than the critical value and B becomes an extorter, warfare ceases and all excess resources stored by A are paid in tribute to B. The technology level of B temporarily stops growing. However, because of high starvation levels in A (due to lack of stored resources) along with technological diffusion from B, A’s technological advancement continues to grow. Eventually, the warfare advantage that B possesses will fall back below the threshold (due to the relative increase in the technological advancement of A) and the two societies will once again go to war. Warfare will jumpstart the technological growth in B and help increase its population advantage as it beats up on A once again pushing the warfare advantage back up towards the threshold value. This process continues ad infinitum.
Intersocietal Dynamics: Five Societies
To study the dynamics of five interacting societies, five single societies were placed in a one-dimensional array, commonly known as a “5-line” topology in network exchange theories. Figure 4 displays the arrangement of the societies. They have been labeled in accordance with Willer’s book Network Exchange Theory. The two societies labeled A will be referred to as the edge societies, the societies labeled B will be referred to as the interstitials, and the society labeled C will be referred to as the center society. Elementary Theory (ET) does not distinguish between the two As and the two Bs because they are treated as identical actors with identical regular positions. Therefore they are indistinguishable (Willer 1999).
Figure 4. Intersocietal 5-Line Network Arrangement

The results of the 5-line intersocietal model are similar to the results of the 2-line model. For low levels of ρ, we see long periods where the populations of the five societies reach stable equilibriums of equal value and hierarchy is absent for long periods. However, for ρ values larger than approximately ρc = 1.5, the system starts in a destabilized state that after a brief transient period reaches a stable hierarchy. When the solution is in a state of equality and also when it is in a stable state of hierarchy, the edge societies are indistinguishable and the interstitial societies are indistinguishable (barring noise). However, when it is in the brief transient destabilized state it acts chaotically, and at times, these nodes differentiate. Because we are only interested in the stable parts of the solution, we stick to the labels provided by ET.
In our intersocietal models, power is defined in terms of population size and warfare advantage. The society with the largest population is getting the most resources out of the system and therefore might be considered the most powerful. However, warfare advantage accounts for population size as well as technological achievement and measures the amount of military force a society has while engaging in coercion and conflict. In both the 2-line and 5-line models, population size and warfare advantage are correlated. This makes it easy to determine power in the intersocietal network. In the 5-line, upon reaching a stable hierarchy, the solutions always settle into the same configuration no matter how the initial population levels are varied. Under certain conditions, it takes longer to reach the final configuration, but the same stable hierarchy is inevitable.
Figure 5. Intersocietal 5-Line Model with ρ = 4
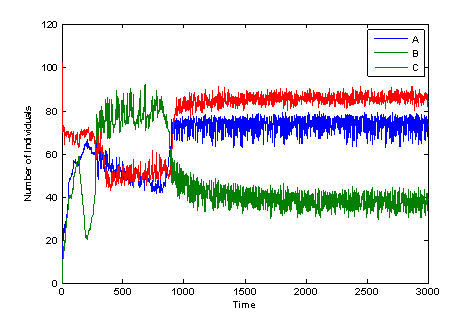
Figure 5 displays the population levels for one of the A nodes, one of the B nodes, and the C node. Again, the A nodes were indistinguishable and the B nodes were indistinguishable (barring noise) so the graph contains all the information necessary to understand the solution. Ultimately, the model can be viewed as a constant battle between two powers; a group containing societies A & C (the edges and the center) vs. a group containing only the two B societies (the interstitials). At first, the interstitial societies, taking the brunt of warfare deaths due to the numbers disadvantage (two vs. three), rapidly decrease in population size. This allows their technology levels to grow at a much faster rate than their opponents’. Because the population growth of the edges and center societies does not compensate for the boost in interstitial technology during this time, the interstitials soon gain enough momentum to capture the overall warfare advantage. However, their time spent as the most powerful nodes is short lived. It is only a matter of time before the numbers catch up to them. Eventually the combined technology level of the edges and center recovers and they overtake the interstitials for good.
Though the final steady state population levels of the edge societies are identical, they do not end up at the same level as the center society. The center society always emerges with the highest population level, followed closely by the edges. The final steady state population levels of the interstitial societies are always much lower. This is true with warfare advantage as well. Therefore, in the 5-line, the center is the most powerful node, followed by the edges, and then the interstitials. This is an important diversion from the expected behavior of a strong-power network, but insights derived from ET that can help to explain why this particular power hierarchy emerges will be discussed later.
Introducing Resource Bias
All nodes in the prior two models were built to be identical with one another. While this limiting assumption allowed for the implications of network size on societal interaction to be most clearly highlighted, it is likely not the case that all societies are privy to identical resource environments. By assigning different resource carrying capacities to the different structural positions in the existing models, we can highlight how heterogeneous enrichment levels interact with topology to produce even more complex network dynamics.
Resource biased 2-line Model
When the carrying capacity of the resources (K) is differentiated so that the environment of one of the two societies is more resource rich than the other, the steady state outcomes are altered, but the forms of the solutions are not. For the cases where ρ < ρc, and the solutions begin with the two populations at stable equilibriums, hierarchy exists from the start as they are no longer at the same population level. The society with a higher resource carrying capacity reaches a higher population level. This in turn influences the outcome of the future hierarchy formed after the system destabilizes. The most powerful society before destabilization remains the most powerful society afterward. When ρ > ρc, and the system starts in a destabilized state, resource bias only affects solutions in which both populations start at the same value. In the unbiased 2-line model, this situation yields solutions where the emergent hegemon is chosen at random with equal odds between the two societies. In the biased model, the society with the resource advantage always emerges as the hegemon. However, when one of the two populations starts at a lower population level the results are unaffected by resource bias. The dynamics discussed in the 2-line section where the society with a lower initial population builds enough momentum from technology and population growth to gain the warfare advantage indefinitely always occurs. This is true even when the society with higher initial population is provided a significantly higher K value.
The reason resource bias does not alter the aforementioned situation is due to the fact that the society with the smaller initial population level (B in our example from earlier) overtakes the other (A) early in the solution, before A can approach its much higher carrying capacity. Once B has overtaken A, it doesn’t matter how many potential resources the environment of A yields. It can never reach carrying capacity for the same reasons it couldn’t in the unbiased model. Every time A grows and starts to gain in military advantage, B resorts to warfare and the population of A is reduced. The environment of A could have an infinite potential for resource production but its low population levels would never get the chance to exploit it.
Resource biased 5-line Model
Due to the differences in network topology, the results of the resource biased 5-line model are different from the 2-line model. It appears that no matter how much bias the B societies (the interstitials) receive, they can never establish themselves as the powerful nodes in the system. Because they possess a large number of warfare partners,, they are always at a disadvantage. Though higher levels of K than their opponents can prolong the early dominance of the interstitials, they can never maintain it. Once they lose power and drop to the bottom of the hierarchy, the potential for higher levels of production becomes irrelevant for the same reasons outlined in the 2-line model. Therefore solutions where the interstitials receive biased enrichment levels reach identical outcomes (barring noise) to the unbiased model.
Biasing the K levels of the center society or the edge societies does impact the long term outcomes. As was discussed earlier, the inevitable hierarchy has the center society just above the edges who are well above the interstitials. When the center society is given a higher K value, it remains the most powerful node, but its population advantage is greater in proportion to the increase in K. Similarly, the steady state population levels of the edge societies grow in proportion to the increase in K bias they receive. As K is increased, they catch up to the center in terms of population and warfare advantage, and eventually become the most powerful nodes in the system at high enough levels of K.
Discussion
Theories of societal evolution are often framed in terms of the endogenous dynamics of a single system (a la Marx), or the evolution of form by competition between a society and its (fixed) environment (a la Spencer). While these are useful approaches at the most abstract level, empirical societies exist in the context of other societies that are also actively changing. That is, to varying degrees, societal evolution is really co-evolution. The exigencies that confront a single society will push that society to adapt to the problems it faces. These changes in a single society have implications for the network that society is embedded in, as this newly adapted society interacts with its neighbors through trade, migration, and warfare. These forces are themselves driving forces in the societal evolution of the other societies in the system. While it is true to state that societies and social forms evolve, it is also true to note that systems evolve, and it is difficult (if not impossible) to abstract the former from the latter. In some cases, the rise of one society is linked to the fall of others; in other cases, upward sweeps or collapse may occur in both.
Our model of the predator-prey relationship between a very small scale human society and its environment enjoys a relatively stable existence. Most historical societies, however, do not exist in inert environments. Rather, they are embedded in world-systems of societies occupying a geographical matrix that structures interactions among them. Our 2-line and 5-line models explore simple world-system networks composed of two populations. Following Hall and Chase-Dunn, we suppose that several forms of relations exist between the societies: migration, warfare, and exploitative trade. Using elementary theory we attempt to categorize these processes.
Elementary Theory argues that there are three types of social relations based on the giving and receiving of sanctions (Willer 1999). When two actors that interact in a network both give and receive positive sanctions, this process is called exchange. When both actors give and receive negative sanctions, it is called conflict. Finally when one actor gives (or threatens) a negative sanction and receives a positive sanction, this is called coercion. Most of the theorizing and research based on ET focuses on exchange. However, all three of these processes occur in the social world and often simultaneously. We believe that all three processes are present in our model.
When a group of individuals migrates from Society A to Society B, it is because the population pressure and living conditions in Society A are worse than in Society B. If the societies are viewed as actors with preference states (like in ET), Society A prefers to yield some warfare advantage to B in exchange for higher average consumption levels. B who is not facing population pressure benefits from receiving the individuals and grows in relative power. Therefore the process of migration in our model might be viewed as the giving and receiving of positive sanctions between actors, and would be classified as exchange.
As discussed earlier in the paragraph describing the trade relations of our model, trade is a coercive process. The threat of force by societies with a warfare advantage to extract a net resource flow in their direction follows the template of coercion. When this process is taken to the extreme, tributary empires are formed whereby the threat of force from one society is exchanged for the entirety of transferrable resources from the other society.
Last, we argue that the process of warfare is a form of conflict, a relatively uncontroversial claim. When engaging in war, both interacting societies suffer the losses of major portions of their population in attempts to establish dominance and achieve power in the network. Both parties receive negative consequences from the interaction, and thus warfare should be classified in ET terms as a form of conflict.
In the single society model, where warfare (conflict) and trade (coercion) are absent, migration (exchange) is an important demographic regulator[1]. However, in the intersocietal models, the role of migration as a regulator decreases, and it turns out that it is the least influential type of social relation between societies. It only occurs in peaceful times and alters an insignificant amount of the relative warfare advantage between societies. Because exchange relations are beneficial to actors, the more interaction partners a society has, the more they can benefit from migration (either by reducing their population pressure when they have excess individuals, or increasing warfare advantage when their partner does). NET predicts that the more exchange partners an actor has relative to others in a given network, the less likely he/she is to be excluded from exchange at any given time, and the more power he/she will have in the network (Willer 1999). However, because migration has such a small impact in our model, we do not necessarily think power is linked to network ties in this manner.
Warfare, on the other hand, is the most important type of social relation in our intersocietal models. Consistent with Chase-Dunn and Hall’s iteration model, warfare is the dominant regulator in these early intersocietal systems. Because warfare is detrimental and not beneficial to actors, a different argument parallel to the one made by NET is should be true. The more conflict partners a society has relative to others in a given network, the less likely it is to be excluded from conflict at any given time, and the less power it will have in the network. In our model, the interstitial societies are never excluded from warfare so they suffer the greatest detriment from these conflictual relations and are always the least powerful actors in the network. The other three societies stand to be excluded from warfare on occasion, and are thus the more powerful actors in the network. However, we also note that the modified argument from NET predicts that the edge societies, who will face the most exclusion on average, should be the most powerful nodes followed closely by the center. This is not what we see. However, conflict is not the only influential type of social relation in our model.
Coercion also plays an important role, especially when the warfare advantage of the more powerful partner crosses the threshold that allows it to become the extorter. The relationship between coercion and power in a network is not as simple as exchange and conflict. If a society is less powerful than most of its coercion partners in a given network, then the more coercion partners it has, the more detriment it receives from interaction and the less powerful it will be. However, if a society is more powerful than most of its coercion partners in a given network, the opposite is true, and more coercion partners implies even more power (due to the exploitation of those partners). This argument is consistent with the results of our model.
The fact that the center and edge societies are excluded from warfare allows them to establish themselves as more powerful than the interstitials. However, once they become the powerful nodes in the system, the more coercion partners that they posses, the more resources they will be able to exploit, and the more powerful their position in the final hierarchy. Because the center society is less likely to be excluded from coercion than the edge societies, and coercion is beneficial to these three powerful societies, the center society gains more from trade and maintains the most power in the network. The center society rides its exclusion from conflict to a powerful position and then adds to that power by not being excluded from coercion.
The results of this model demonstrate how important network analysis can be when studying the macro social world. The success of societies in a system is influenced by the behavior of every other society in the system. As was shown in the 5-line model, network structure often trumps other predictors of success such as the enrichment levels of a society’s environment. Regardless of the value of K, the interstitial societies can never escape their poor network position. Macro historical researchers would be wise to incorporate theories from ET and other network theories into their analyses of world power distributions and hierarchies.
Conclusions
The models and results above are no more (or less) than a demonstration that some patterns of population oscillation and hierarchy formation observed by researchers on the histories of world systems of societies could be driven by relatively simple dynamic processes within and between societies located on geographical networks. The rise and fall of human settlements, the alteration between periods of chronic warfare and periods of peace, the emergence of hierarchies both stable and unstable across societies can all result from the simulation of very simple world systems. That such systems can generate such dynamics, of course, is not proof that they did generate such dynamics.
There is much work to be done with the very simple model used here. A line network of five nodes can produce interesting and non-obvious behavior. But empirical systems have more complicated and non-regular topologies. As travel and communication technologies grow, for example, societies may learn how to bypass one another to communicate or trade with more distant neighbors. While not simulated here, our results suggest that it is not easy to anticipate what forms of order might emerge from different networks with different, perhaps even evolving, topologies.
The current model envisions very small societies with minimal technology – material, cultural, or social organizational. The societies in our model do, in the face of selection pressures, develop greater complexity. And, although we have not focused on these dynamics in this report, uneven technological development is one source of the systemic dynamics reported here. It is important to note, however, that our technological development functions are smooth and slow. The sources and consequences of major leaps forward (like the domestication of new plant or animal species, the adaptation of the wheel for use in transportation, etc.) for the patterns of order in world systems is a fertile area for further work.
Also, our model focuses almost entirely on the formation of stable hierarchies between societies, rather than within them. Absent from this examination are many factors crucial to understanding the development of human societies, including the formation of culture, the differentiation of the basic human institutions, and the division of labor as technology and population grow. While these elements were left out to highlight the importance of intersocietal dynamics, further simulations should spend greater focus on the internal dynamics of a society that extend beyond mere resource acquisition.
References
Bang, Peter Fibiger (ed.) 2003. "Tributary Empires in History: Comparative Perspectives from Antiquity to the Late Medieval" The Medieval History Journal, 6(2).
Barro, Robert J. and Xavier Sala-i-Martin. 1997. "Technological Diffusion, Convergence, and Growth." Journal of Economic Growth 2:1-26.
Berryman, Alan. 1992. “The Origins and Evolution of Predator-Prey Theory.” Ecology, 73(5):1530-1535
Carneiro, Robert L. 1970. "A Theory of the Origin of the State." Science 169:733-738.
Chase-Dunn, Christopher. 2001. "World Systems Theorizing." Pp. 589-612 in Handbook of Sociological Theory, edited by J. H. Turner. New York: Springer.
Chase-Dunn, Christopher and Peter Grimes. 1995. "World-Systems Analysis." Annual Review of Sociology 21:387-417.
Chase-Dunn, Christopher and Thomas D. Hall. 1997. Rise and Demise: Comparing World Systems. Boulder, CO: Westview Press.
Harris, Marvin. 1977. Cannibals and Kings: The Origins of Cultures. New York: Random House.
Kottak, Conrad P. 1972. "Ecological Variables in the Origin and Evolution of African States: The Buganda Example." Comparative Studies in Society and History 14:351-380.
Maloney, Sean M. 2008. "A Violent Impediment: The Evolution of Insurgent Operations in Kandahar Province 2003-2007." Small Wars and Insurgencies 19:201-220.
Parsons, Talcott. 1982. On Institutions and Social Evolution. Chicago: University of Chicago Press.
Rosenzweig, Michael. 1971. “Paradox of Enrichment: Destabilization of Exploitation Ecosystems in Ecological Time.” Science, 171(3969):385-387
Spencer, Herbert and Robert L. Carneiro. 1967. The Evolution of Society: Selections from Herbert Spencer's Principles of Sociology. Chicago, IL: University of Chicago Press.
Strogatz, Steven Henry and Ian Stewart. 1993. "Coupled Oscillators and Biological Synchronization." Scientific American December:102-109.
Tagliacozzo, Eric. 2007. "An Urban Ocean: Notes on the Historical Evolution of Coastal Cities in Greater Southeast Asia." Journal of Urban History 33:911-932.
Tainter, Joseph. 1988. The Collapse of Complex Societies. Cambridge, MA: Cambridge Press.
Turchin, Peter. 2003. Complex Population Dynamics. Princeton, NJ: Princeton University Press.
Watts, Duncan J. 1999a. "Networks, Dynamics, and the Small-World Phenomenon." American Journal of Sociology 105:493-527.
—. 1999b. Small Worlds: The Dynamics of Networks between Order and Randomness. Princeton, NJ: Princeton University Press.
Willer, David. 1999. "Network exchange theory: Issues and directions." Pp. 1-22 in Network Exchange Theory, edited by D. Willer. Westport, CT: Praeger.